Pressure drop reduction in corrugated pipe, and flow behavior of solid nitrogen particles

　With respect to corrugated pipe, studies have been conducted by numerous researchers on pressure drop. However, most of these involve water or air at room temperature. The lengths of corrugated pipe in actual use are also typically short, and based on experience, designs have tended to assume that pressure drop will correspond to that in smooth-walled pipe having 3-4 times the length.

　Corrugated pipes serving to absorb pipe contraction at cryogenic temperatures are essential elements of piping arrangements for cryogenic equipment. Increased pressure drop in piping necessitates greater required power for transport pumps, and is a cause of decreased system performance. In the case of stainless steel, for example, cooling from room temperature to cryogenic levels (77 K, 20 K, or 4 K) results in pipe contraction of approximately 3 mm per 1 m in pipe length. Accordingly, substantial stresses are placed on piping and equipment, which can easily lead to damage. In the case of the long-distance transport piping system, the total length of corrugated pipe would be substantial with pressure drop properties taking on major importance.

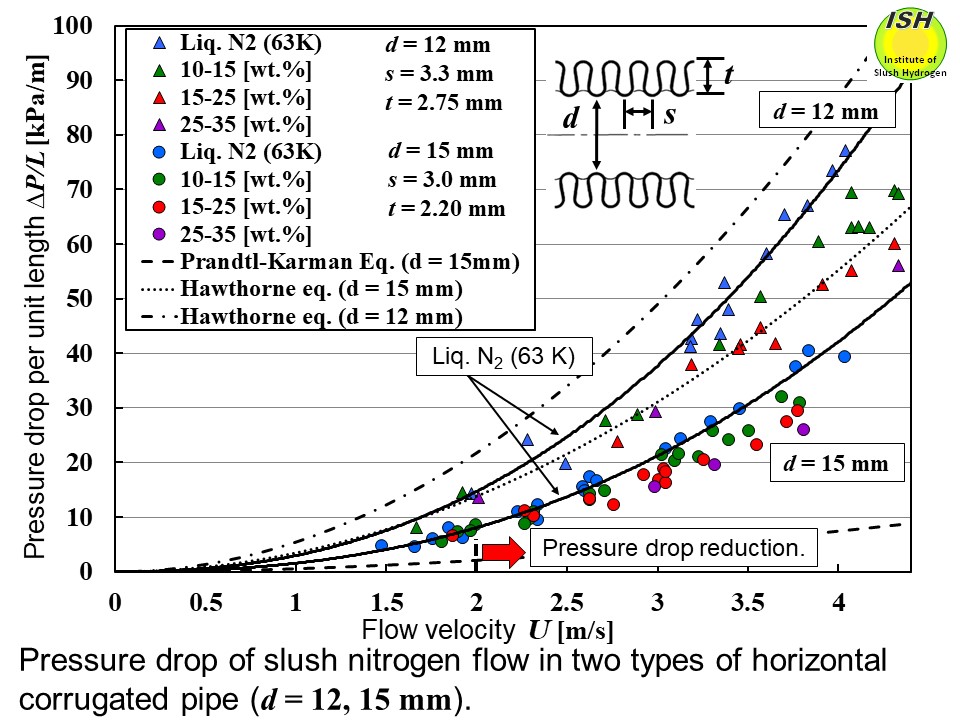
　Measurement results for flow velocity and pressure drop per unit length in two types of horizontal annular (not spiral) type corrugated pipe with inner diameters d = 12 mm (Type A) and d = 15 mm (Type B) are illustrated in the above figure [28]. The measured values for liquid nitrogen, the least squares approximation curves, the Prandtl-Karman eq. and the Hawthorne eq. (A: λ = 0.151, B: λ = 0.120) are shown. Hawthorne et al. [34] have reported a pressure drop eq. for water and air at room temperature in corrugated pipe.
　At the same flow velocity, pressure drop values for liquid nitrogen in the corrugated pipes A and B are 5-9 and 3-6 times the Prandtl-Karman eq. values, respectively. All of the values calculated for liquid nitrogen pressure drop using the Hawthorne eq. were greater than the experimental values. With respect to slush nitrogen pressure drop in the two corrugated pipes, the values were about the same or slightly higher in both cases than for liquid nitrogen in the low flow velocity region of within 2 m/s, while pressure drop reduction was exhibited in the region above 2 m/s, with the amount of reduction becoming greater as the solid fraction increased.
　The maximum amounts of pressure drop reduction (%) obtained in the experiment, together with the solid fractions, were 37% at 30 wt.% for Type A and 31% at 27 wt.% for Type B, as compared to liquid nitrogen.

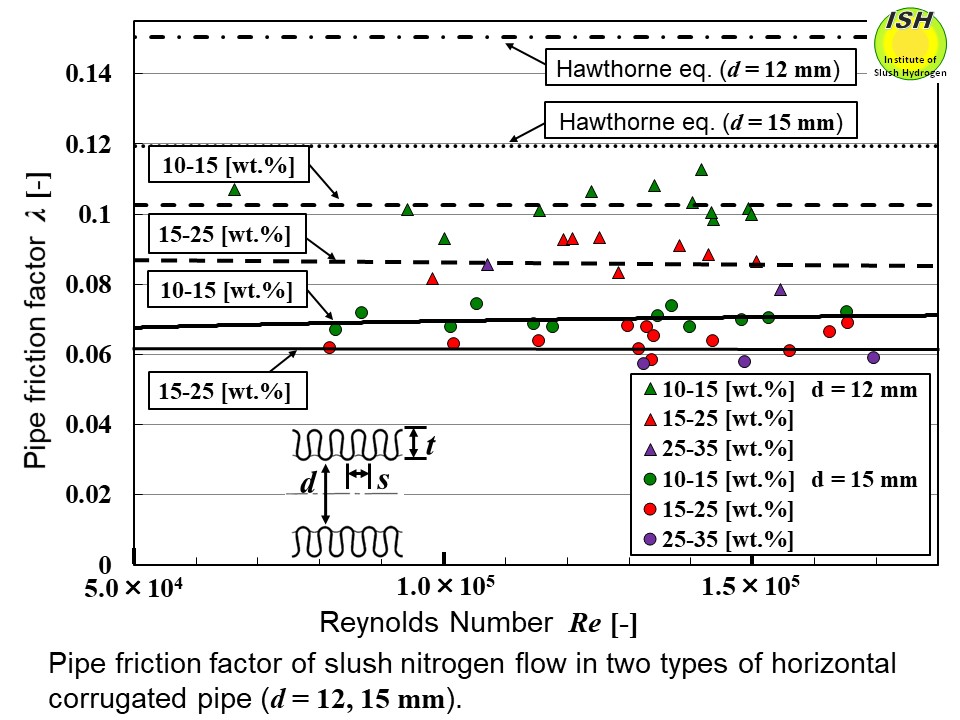
　The experimental results with respect to the pipe friction factor λ and the Re number for slush nitrogen are given in the figure below [28]. In the figure, the least squares approximation curves corresponding to the experimental values for the solid fraction ranges of 10-15 and 15-25 wt.% are indicated by dashed (Type A) and solid (Type B) lines, respectively. For reference, the Hawthorne eq. values (A: λ = 0.151, B: 0.120) are also given. In the cases of both corrugated pipes A and B, the pipe friction factor for slush nitrogen is nearly a constant value. A clear difference can be observed with respect to low solid fraction (10-15 wt.%) and high solid fraction (15-35 wt.%), with the pipe friction factor becoming lower as the solid fraction increases.

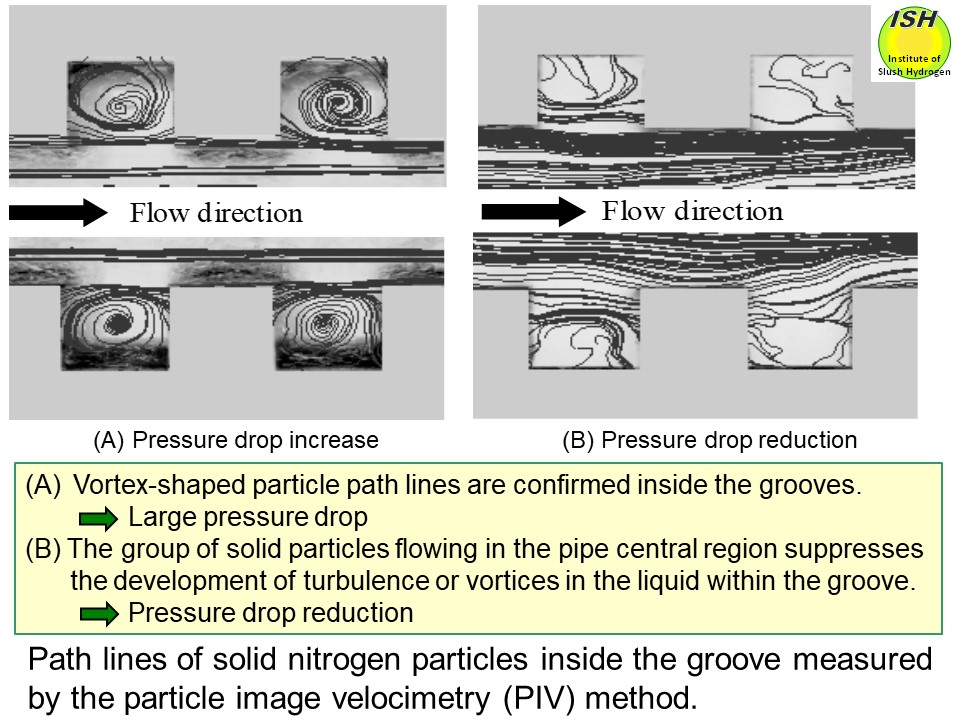
　To clarify the mechanism involved in pressure drop reduction, path lines of solid nitrogen particles inside the transparent grooved rectangular pipe (16 mm×12 mm, with groove depth of 2 mm) to simulate corrugated pipe were measured using the PIV method*.

　The figures below present the visualization of solid nitrogen particles inside the groove taken using a high-speed video camera and the PIV measurement results corresponding to experimental point where pressure drop reduction occurs, and experimental point where reduction does not occur, respectively [28]. Although vortex-shaped particle paths were confirmed within the grooves when the pressure drop reduction did not occur, this was not the case (vortex-shaped particle paths were not confirmed) when the pressure drop reduction occurred. It is considered that the liquid vortex (recirculation) occurrence within the grooves was accompanied by the captured solid particles, causing the loss of kinetic energy with respect to both the liquid and the solid particles, and resulting in increased pressure drop.
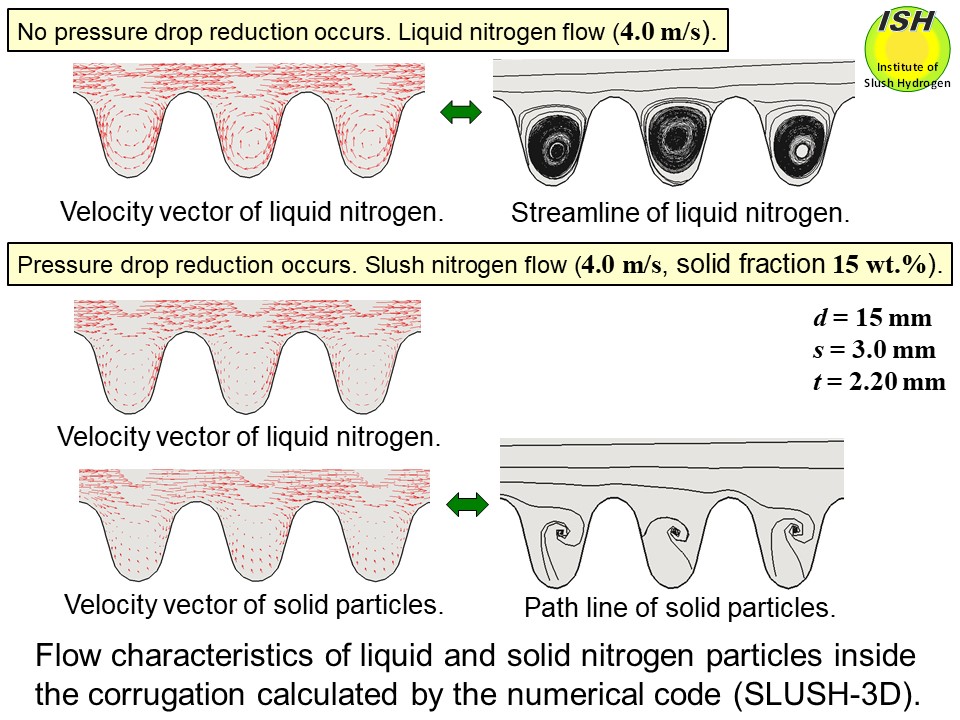
　With the increased flow velocity described in the smooth circular pipe "Circular pipe flow and PIV", the solid particles tended to move towards the pipe center region. As a result, a liquid layer with few solid particles was formed in the vicinity of the corrugation, serving to reduce the interference between the corrugation and the solid particles. Furthermore, as shown in the figure below calculated by the numerical code SLUSH-3D [35], it is considered that the group of solid particles flowing at the pipe center region served to limit the development of turbulence or vortices in the liquid within the corrugation, as well as limiting turbulence diffusion towards the pipe center, thus reducing the loss of kinetic energy from the liquid and the solid particles.

　* PIV method: The velocity of solid particles is directly measured using the Particle Image Velocimetry method.